www.vorhilfe.de
Vorhilfe

Kostenlose Kommunikationsplattform für gegenseitige Hilfestellungen.
Hallo Gast! [ einloggen | registrieren ]
Startseite · Forum · Wissen · Kurse · Mitglieder · Team · Impressum
Forenbaum
^ Forenbaum
Status Englisch
  Status Grammatik
  Status Lektüre
  Status Korrekturlesen
  Status Übersetzung
  Status Sonstiges (Englisch)

Gezeigt werden alle Foren bis zur Tiefe 2

Navigation
 Startseite...
 Neuerdings beta neu
 Forum...
 vorwissen
   Einstieg
   
   Index aller Artikel
   
   Hilfe / Dokumentation
   Richtlinien
   Textgestaltung
 vorkurse...
 Werkzeuge...
 Nachhilfevermittlung beta...
 Online-Spiele beta
 Suchen
 Verein...
 Impressum
Das Projekt
Server und Internetanbindung werden durch Spenden finanziert.
Organisiert wird das Projekt von unserem Koordinatorenteam.
Hunderte Mitglieder helfen ehrenamtlich in unseren moderierten Foren.
Anbieter der Seite ist der gemeinnützige Verein "Vorhilfe.de e.V.".
Partnerseiten
Weitere Fächer:

Open Source FunktionenplotterFunkyPlot: Kostenloser und quelloffener Funktionenplotter für Linux und andere Betriebssysteme
Ableitungsregel
Mach mit! und verbessere/erweitere diesen Artikel!
Artikel • Seite bearbeiten • Versionen/Autoren

Ableitungsregel

Satz Ableitungsregeln

Die wichtigsten Ableitungsregeln im Überblick

Potenzregel

$ f(x)=x^n $
$ \Rightarrow\ f'(x)=n\cdot{}x^{n-1} $

Summenregel

$ f(x)=g(x)+h(x) $
$ \Rightarrow\ f'(x)=g'(x)+h'(x) $

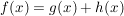
("Die Ableitung einer Summe ist die Summe der Ableitungen.")

Faktorregel

$ f(x)=k\cdot{}g(x) $
$ \Rightarrow\ f'(x)=k\cdot{}g'(x) $

Differenzregel
(Diese Regel ist nicht geläufig, da sie unmittelbar aus Summen- und Faktorregel folgt.)

$ f(x)=g(x)-h(x) $
$ \Rightarrow\ f'(x)=g'(x)-h'(x) $

Produktregel

$ f(x)=g(x)\cdot{}h(x) $
$ \Rightarrow\ f'(x)=g'(x)\cdot{}h(x)+g(x)\cdot{}h'(x) $

Quotientenregel

$ f(x)=\bruch{g(x)}{h(x)} $
$ \Rightarrow\ f'(x)=\bruch{g'(x)\cdot{}h(x)-g(x)\cdot{}h'(x)}{(h(x))^2} $

Kettenregel

$ f(x)=g(h(x)) $
$ \Rightarrow\ f'(x)=h'(x)\cdot{}g'(h(x)) $
("Innere Ableitung mal äußere Ableitung.")


Umkehrregel für Umkehrfunktionen:

$ f^{-1}(y) $ sei die Umkehrfunktion zu y=f(x).
Dann gilt:
$ (f^{-1})'(f(x))=\frac{1}{f'(x)} $ oder $ (f^{-1})'(f(x))\cdot{}f'(x)=1 $





Bemerkungen.

TODO


Beispiele.


Beweis.

Die Beweise finden sich in den einzelnen Artikeln zu den Ableitungsregeln.

Erstellt: Sa 04.09.2004 von Marc
Letzte Änderung: Mi 25.11.2009 um 18:47 von informix
Weitere Autoren: dietlind
Artikel • Seite bearbeiten • Versionen/Autoren • Titel ändern • Artikel löschen • Quelltext
^ Seitenanfang ^
www.englischraum.de
[ Startseite | Forum | Wissen | Kurse | Mitglieder | Team | Impressum ]